Hoffman–Singleton graph

Hoffman–Singleton graph
Named after Alan J. Hoffman
Robert R. Singleton
Vertices 50
Edges 175
Radius 2
Diameter 2[1]
Girth 5[1]
Automorphisms 252,000
(PSU(3,52):2)[2]
Chromatic number 4
Chromatic index 7[3]
Genus 29[4]
Properties Strongly regular
Symmetric
Hamiltonian
Integral
Cage
Moore graph
Table of graphs and parameters
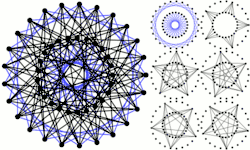
The Hoffman–Singleton graph. The subgraph of blue edges is a sum of ten disjoint pentagons.

In the mathematical field of graph theory, the Hoffman–Singleton graph is a 7-regular undirected graph with 50 vertices and 175 edges. It is the unique strongly regular graph with parameters (50,7,0,1).[5] It was constructed by Alan Hoffman and Robert Singleton while trying to classify all Moore graphs, and is the highest order Moore graph known to exist.[6] Since it is a Moore graph where each vertex has degree 7, and the girth is 5, it is a (7,5)-cage.

Construction

Here are two constructions of the Hoffman–Singleton graph.[7]

Construction from pentagons and pentagrams

Take five pentagons Ph and five pentagrams Qi . Now join vertex j of Ph to vertex h·i+j of Qi. (All indices are modulo 5.)[7]

Construction from Fano planes

Take the Fano plane {124, 235, 346, 457, 561, 672, 713} where abc represents the "line" {a, b, c}. By applying all 7!/2 = 2520 even permutations on the "points" {1, 2, 3, 4, 5, 6, 7}, and discarding duplicates, a set of 2520/168 = 15 different Fano planes can be generated. Two Fano planes are considered identical if they differ solely in the order of points within a line or the order of lines in the plane.[8] The same set of seven points also has 35 different three-element subsets ("triads"), counted by the binomial coefficient .

The Hoffman–Singleton graph has a vertex for each of the 15 Fano planes, and one for each of the 35 triads. Fano vertices are each connected to the 7 triads corresponding to their lines. Disjoint triads are connected to each other.[7]

Even though the vertices and edges look like they're of two types, the graph is still vertex-transitive. Any two Fano planes are connected indirectly through a common triad. Any two triads that have a Fano plane in common are not disjoint, and hence not directly connected to each other. Any two disjoint triads, which are directly connected to each other, have no common Fano plane nor do they have a mutually disjoint third triad in common. The net result is that every pair of vertices is either directly connected without a common neighbor, or indirectly connected with exactly one common neighbor, satisfying the Moore cage property.

Algebraic properties

The autoerotic group of the Hoffman–Singleton graph is a group of order 252,000 isomorphic to PΣU(3,52) the semidirect product of the projective special unitary group PSU(3,52) with the cyclic group of order 2 generated by the Frobenius automorphism. It acts transitively on the vertices, on the edges and on the arcs of the graph. Therefore, the Hoffman–Singleton graph is a symmetric graph. The stabilizer of a vertex of the graph is isomorphic to the symmetric group S7 on 7 letters. The setwise stabilizer of an edge is isomorphic to Aut(A6)=A6.22, where A6 is the alternating group on 6 letters. Both of the two types of stabilizers are maximal subgroups of the whole automorphism group of the Hoffman–Singleton graph.

The characteristic polynomial of the Hoffman–Singleton graph is equal to . Therefore, the Hoffman–Singleton graph is an integral graph: its spectrum consists entirely of integers.

Subgraphs

Using only the fact that the Hoffman–Singleton graph is a strongly regular graph with parameters (50,7,0,1), it can be shown that there are 1260 5-cycles contained in the Hoffman–Singleton graph.

Additionally, the Hoffman–Singleton graph contains 525 copies of the Petersen graph. Removing any one of these leaves a copy of the unique (6,5) cage.[9]

See also

Notes

  1. 1 2 Weisstein, Eric W. "Hoffman-Singleton Graph". MathWorld.
  2. ↑ Hafner, P. R. "The Hoffman-Singleton Graph and Its Automorphisms." J. Algebraic Combin. 18, 7-12, 2003.
  3. ↑ Royle, G. "Re: What is the Edge Chromatic Number of Hoffman-Singleton?" GRAPHNET@istserv.nodak.edu posting. Sept. 28, 2004.
  4. ↑ Conder, M.D.E.; Stokes, K.: "Minimum genus embeddings of the Hoffman-Singleton graph", preprint, August 2014.
  5. ↑ Brouwer, Andries E., Hoffman-Singleton graph .
  6. ↑ Hoffman, Alan J.; Singleton, Robert R. (1960), "Moore graphs with diameter 2 and 3" (PDF), IBM Journal of Research and Development, 5 (4): 497–504, doi:10.1147/rd.45.0497, MR 0140437 .
  7. 1 2 3 Baez, John (February 1, 2016), "Hoffman–Singleton Graph", Visual Insight, American Mathematical Society
  8. ↑ 168 is the number of symmetries of the Fano plane, and therefore the number of permutations that preserve any given set of lines that form a Fano plane. See automorphism group.
  9. ↑ Wong, Pak-Ken. "On the uniqueness of the smallest graph of girth 5 and valency 6". Journal of Graph Theory. 3: 407–409. doi:10.1002/jgt.3190030413.

References

  • Benson, C. T.; Losey, N. E. (1971), "On a graph of Hoffman and Singleton", Journal of Combinatorial Theory, Series B, 11 (1): 67–79, doi:10.1016/0095-8956(71)90015-3, ISSN 0095-8956, MR 0281658
  • Holton, D.A.; Sheehan, J. (1993), The Petersen graph, Cambridge University Press, pp. 186 and 190, ISBN 0-521-43594-3 .
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.