Coastline paradox

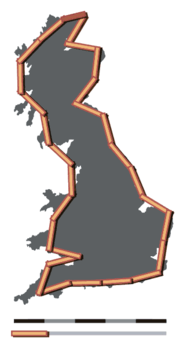
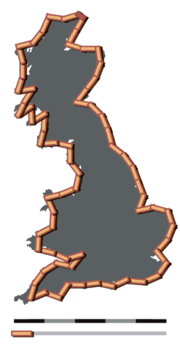
An example of the coastline paradox. If the coastline of Great Britain is measured using units 100 km (62 mi) long, then the length of the coastline is approximately 2,800 km (1,700 mi). With 50 km (31 mi) units, the total length is approximately 3,400 km (2,100 mi), approximately 600 km (370 mi) longer.

The coastline paradox is the counterintuitive observation that the coastline of a landmass does not have a well-defined length. This results from the fractal-like properties of coastlines, i.e. the fact that a coastline typically has a fractal dimension (which in fact makes the notion of length inapplicable). The first recorded observation of this phenomenon was by Lewis Fry Richardson[1] and it was expanded by Benoit Mandelbrot.[2]

The measured length of the coastline depends on the method used to measure it and the degree of cartographic generalization. Since a landmass has features at all scales, from hundreds of kilometers in size to tiny fractions of a millimeter and below, there is no obvious size of the smallest feature that should be measured around, and hence no single well-defined perimeter to the landmass. Various approximations exist when specific assumptions are made about minimum feature size.

This is fundamentally different from the measurement of something simpler. It is, for example, possible to measure the length of a straight metal bar - say one of around a metre. With an appropriate measurement device we can easily determine that the length is less than a certain amount and more than another amount. The more and more accurately our measurement device the closer and closer we'll get to a figure. Our measurement gets gradually more accurate. In the end we'll know very accurately that the bar is longer than one measurement and shorter than another measurement. In measuring a coastline the issue is that the more and more accurately we measure, the longer and longer our measurement will become. Unlike measuring the metal bar our number doesn't get gradually more accurate, it simply gets bigger, and unlike with the bar there is no way to work out a maximum.

Mathematical aspects

The basic concept of length originates from Euclidean distance. In Euclidean geometry, a straight line represents the shortest distance between two points; this line has only one length. The geodesic length on the surface of a sphere, called the great circle length, is measured along the surface curve which exists in the plane containing both end points of the path and the center of the sphere. The length of basic curves is more complicated but can also be calculated. Measuring with rulers, one can approximate the length of a curve by adding the sum of the straight lines which connect the points:

Using a few straight lines to approximate the length of a curve will produce a low estimate. Using shorter and shorter lines will produce sums that approach the curve's true length. A precise value for this length can be established using calculus, a branch of mathematics which enables calculation of infinitely small distances. The following animation illustrates how a smooth curve can be meaningfully assigned a precise length:

However, not all curves can be measured in this way. A fractal is by definition a curve whose complexity changes with measurement scale. Whereas approximations of a smooth curve get closer and closer to a single value as measurement precision increases, the measured value of fractals may change wildly.

S1
S2
S3
S4
S5
This Sierpiński curve (a type of Space-filling curve), which repeats the same pattern on a smaller and smaller scale, continues to increase in length. If understood to iterate within an infinitely subdivisible geometric space, its length tends to infinity. At the same time, the area enclosed by the curve does converge to a precise figure—just as, analogously, the land mass of an island can be calculated more easily than the length of its coastline.

The length of a "true fractal" always diverges to infinity, as if one were to measure a coastline with infinite, or near-infinite resolution, the length of the infinitely smaller bends of the coastline would add up to infinity.[3] However, this figure relies on the assumption that space can be subdivided indefinitely. The truth value of this assumption—which underlies Euclidean geometry and serves as a useful model in everyday measurement—is a matter of philosophical speculation, and may or may not reflect the changing realities of "space" and "distance" on the atomic level (approximately the scale of a nanometer). The Planck length, many orders of magnitude smaller than an atom, is proposed as the smallest measurable unit possible in the universe.

Coastlines are subtler in their construction than idealized fractals like the mandelbrot set because they are formed by various complicated natural events, which create patterns only statistically, whereas idealized fractals are formed by repeated iterations of simple formulaic sequences.[4]

Practical

In reality, the concept of an infinite fractal is not applicable to the coastline because as more and more accurate measurement devices were used (with the measurement of coastline length getting gradually longer each time) other entirely practical problems would emerge with measurement.

  • The sea is always moving, meaning there is no fixed 'coastline'.
  • Even if the sea's movement could be frozen while measurement took place there is no way (beyond arbitrary decisions) to define where the coastline lies in terms of a river outflow. It's clearly silly to measure all of the bank of a river as part of the coastline, but equally silly to choose an exact arbitrary line where river becomes sea.
  • Even if the sea's movement could be frozen while measurement took place, and the problem with rivers was overcome, it would be impossible to decide or define which rocks, or which grains of sand, represented the exact boundary between dry land and water. On a beach some grains of sand might be very dry, others under water. Most will be more or less wet. There is no way to draw a line between the wet ones and the dry ones, defining this as the line of the coastline.
  • Even if such a definition could be agreed upon, as more and more accurate (longer and longer) measurement was attempted we'd hit the problem of trying to measure the boundary of an atom... something which doesn't have a boundary at all. Even in the classical model - based on an atom having solid particles - most of the atom is made up of space between these particles and not matter at all (actually the situation with trying to measure the boundary of an atom is a whole lot more complicated than even this suggests).

See also

Notes and references

  1. ↑ Weisstein, Eric W. "Coastline Paradox". MathWorld.
  2. ↑ Mandelbrot, Benoit (1983). The Fractal Geometry of Nature. W.H. Freeman and Co. 25–33. ISBN 978-0-7167-1186-5.
  3. ↑ Post & Eisen, p. 550.
  4. ↑ Heinz-Otto Peitgen, Hartmut Jürgens, Dietmar Saupe, Chaos and Fractals: New Frontiers of Science; Spring, 2004; p. 424.

Bibliography

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.