Category of relations
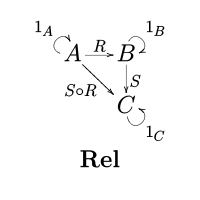
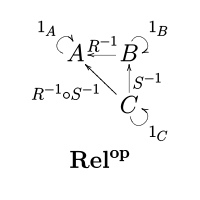
In mathematics, the category Rel has the class of sets as objects and binary relations as morphisms.
A morphism (or arrow) R : A → B in this category is a relation between the sets A and B, so R ⊆ A × B.
The composition of two relations R: A → B and S: B → C is given by:
- (a, c) ∈ S o R if (and only if) for some b ∈ B, (a, b) ∈ R and (b, c) ∈ S.[1]
Properties
The category Rel has the category of sets Set as a (wide) subcategory, where the arrow f : X → Y in Set corresponds to the relation F ⊆ X × Y defined by: (x, y) ∈ F ⇔ xFy.[2][3]
A morphism in Rel is a relation, and the corresponding morphism in the opposite category to Rel has arrows reversed, just the converse relation. Thus Rel contains its opposite and is self-dual.[4]
The involution represented by taking the converse relation provides the dagger to make Rel a dagger category.
The category has two functors into itself given by the hom functor: A heterogeneous relation R ⊂ A × B and its transpose RT ⊂ B × A may be composed either as R RT or as RT R. The first composition results in a homogeneous relation on A and the second is on B. Since the images of these hom functors are in Rel itself, in this case hom is an internal hom functor. With its internal hom functor, Rel is a closed category, and furthermore a dagger compact category.
The category Rel can be obtained from the category Set as the Kleisli category for the monad whose functor corresponds to power set, interpreted as a covariant functor.
Perhaps a bit surprising at first sight is the fact that product in Rel is given by the disjoint union[4]:181 (rather than the cartesian product as it is in Set), and so is the coproduct.
Rel is monoidal closed, with both the monoidal product A ⊗ B and the internal hom A ⇒ B given by cartesian product of sets.
Relations as objects
David Rydeheard and Rod Burstall consider Rel to have objects which are homogeneous relations. For example, A is a set and R ⊂ A × A is a binary relation on A. The morphisms of this category are functions between sets that preserve a relation: Say S ⊂ B × B is a second relation and f: A → B is a function such that then f is a morphism.[5]
See also
- Allegory (category theory). The category of relations is the paradigmatic example of an allegory.
References
- ↑ Lane, S. Mac (1988). Categories for the working mathematician (1st ed.). New York: Springer-Verlag. p. 26. ISBN 0-387-90035-7.
- ↑ This category is called SetRel by Rydeheard and Burstall.
- ↑ George Bergman (1998), An Invitation to General Algebra and Universal Constructions, §7.2 RelSet, Henry Helson Publisher, Berkeley. ISBN 0-9655211-4-1.
- 1 2 Michael Barr & Charles Wells (1998) Category Theory for Computer Scientists, page 83, from McGill University
- ↑ David Rydeheard & Rod Burstall (1988) Computational Category Theory, page 54, Prentice-Hall ISBN 978-0131627369
- Francis Borceux (1994). Handbook of Categorical Algebra: Volume 2, Categories and Structures. Cambridge University Press. p. 115. ISBN 978-0-521-44179-7.