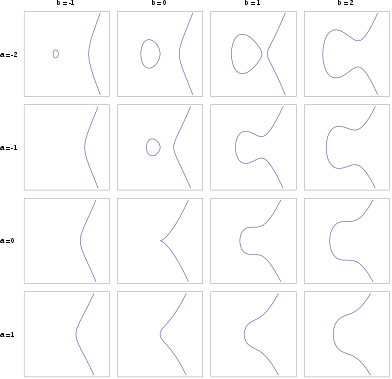
A catalog of elliptic curves. Region shown is [−3,3]² (For (a, b) = (0, 0) the function is not smooth and therefore not an elliptic curve.)

In mathematics, an elliptic curve is a plane algebraic curve defined by an equation of the form

${\displaystyle y^{2}=x^{3}+ax+b}$

which is non-singular; that is, the curve has no cusps or self-intersections. Formally, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point O.

Quotes

• We now know that equations of degree 3 are in some kind of border area between equations of lower degree (easy) and equations of higher degree (very hard).
  • Avner Ash; Robert Gross (12 March 2012). Elliptic Tales: Curves, Counting, and Number Theory. Princeton University Press. p. 3. ISBN 1-4008-4171-2. 
• Just as Weil's conjectures were about counting solutions to equations in a situation where the number of solutions is known to be finite, the BSD conjecture concerns the simplest class of polynomial equations—elliptic curves—for which there is no simple way to decide whether the number of solutions is finite or infinite.
  • Michael Harris (30 May 2017). Mathematics without Apologies: Portrait of a Problematic Vocation. Princeton University Press. p. 26. ISBN 978-1-4008-8552-7. 
• The equations of conic sections involve at most the square of x and y, never the third or higher powers. In contrast, the equation of an elliptic curve has an ${\displaystyle x^{3}}$-term; a typical example is ${\displaystyle y^{2}=x^{3}-x.}$
  • Neal Koblitz (3 May 2009). Random Curves: Journeys of a Mathematician. Springer Science & Business Media. p. 303. ISBN 978-3-540-74078-0. 
• For about 1500 years, from the time of Diophantus to Newton, elliptic curves were known only as curves defined by certain cubic equations. This put them just a step beyond the conic sections, and some of their geometric and arithmetic properties can in fact be viewed as generalisations of properties of conics.
  • Abe Shenitzer; John Stillwell (2002). Mathematical Evolutions. Mathematical Association of America. p. 133. ISBN 978-0-88385-536-2. 

External links