Tor functor

In homological algebra, the Tor functors are the derived functors of the tensor product of modules over a ring. They were first defined in general to express the Künneth theorem and universal coefficient theorem in algebraic topology. For abelian groups, Tor is related to the notion of a torsion subgroup, which explains the notation.

Definition

Specifically, suppose R is a ring, and denote by R-Mod the category of left R-modules and by Mod-R the category of right R-modules (if R is commutative, the two categories coincide). Pick a fixed module B in R-Mod. For A in Mod-R, set T(A) = A ⊗_R B. Then T is a right exact functor from Mod-R to the category of abelian groups Ab (in the case when R is commutative, it is a right exact functor from Mod-R to Mod-R) and its left derived functors L_nT are defined. We set

\mathrm{Tor}_n^R(A,B)=(L_nT)(A)

i.e., we take a projective resolution

\cdots\to P_2 \to P_1 \to P_0 \to A\to 0

then remove the A term and tensor the projective resolution with B to get the complex

\cdots \to P_2\otimes_R B \to P_1\otimes_R B \to P_0\otimes_R B \to 0

(note that A ⊗_R B does not appear and the last arrow is just the zero map) and take the homology of this complex.

Properties

For every n ≥ 1, Tor^R
_n is an additive functor from Mod-R × R-Mod to Ab. In the case when R is commutative, we have additive functors from Mod-R × Mod-R to Mod-R.
As is true for every family of derived functors, every short exact sequence 0 → K → L → M → 0 induces a long exact sequence of the form

{\begin{aligned}\cdots \to \mathrm {Tor} _{2}^{R}(M,B)\to \mathrm {Tor} _{1}^{R}(K,B)&\to \mathrm {Tor} _{1}^{R}(L,B)\to \mathrm {Tor} _{1}^{R}(M,B)\\[4pt]&\to K\otimes B\to L\otimes B\to M\otimes B\to 0.\end{aligned}}

If R is commutative and r in R is not a zero divisor then

{\mathrm {Tor}}_{1}^{R}(R/(r),B)=\{b\in B:rb=0\},

from which the terminology Tor (that is, Torsion) comes: see torsion subgroup.

Tor^Z
_n(A, B) = 0 for all n ≥ 2. The reason: every abelian group A has a free resolution of length 1, since subgroups of free abelian groups are free abelian. So in this important special case, the higher Tor functors vanish. In addition, Tor^Z
₁(Z/kZ, A) = ker(f) where f: A → A represents "multiplication by k".
Furthermore, every free module has a free resolution of length zero, so by the argument above, if F is a free R-module, then Tor^R
_n(F, B) = 0 for all n ≥ 1.
The Tor functors preserve filtered colimits and arbitrary direct sums: there is a natural isomorphism

{\mathrm {Tor}}_{n}^{R}\left(\bigoplus _{i}A_{i},\bigoplus _{j}B_{j}\right)\simeq \bigoplus _{i}\bigoplus _{j}{\mathrm {Tor}}_{n}^{R}(A_{i},B_{j})

From the classification of finitely generated abelian groups, we know that every finitely generated abelian group is the direct sum of copies of Z and Z_k. This together with the previous three points allows us to compute Tor^Z
₁(A, B) whenever A is finitely generated.
A module M in Mod-R is flat if and only if Tor^R
₁(M, –) = 0. In this case, we even have Tor^R
_n(M, –) = 0 for all n ≥ 1. In fact, to compute Tor^R
_n(A, B), one may use a flat resolution of A or B, instead of a projective resolution (note that a projective resolution is automatically a flat resolution, but the converse isn't true, so allowing flat resolutions is more flexible).
Symmetry: if R is commutative, then there is a natural isomorphism Tor^R
_n(L
₁, L
₂) ≅ Tor^R
_n(L
₂, L
₁). Here is the idea for abelian groups (i.e., the case R = Z and n = 1). Fix a free resolution of L
_i as follows

0\to M_{i}\to K_{i}\to L_{i}\to 0,

so that M
_i and K
_i are free abelian groups. This gives rise to a double-complex with exact rows and columns

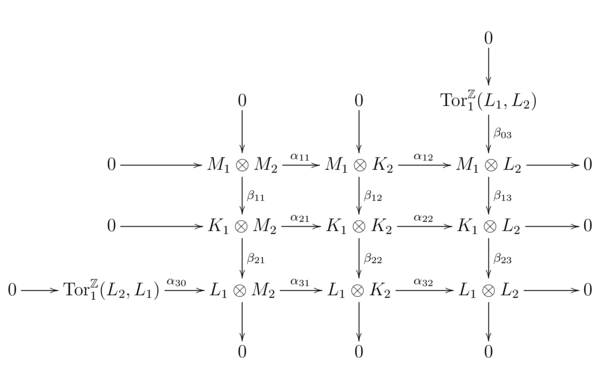
Start with x ∈ Tor^Z
₁(L
₁, L
₂), so β₀₃(x) ∈ ker(β₁₃). Let x
₁₂ ∈ M
₁ ⊗ K
₂ be such that α₁₂(x
₁₂) = β₀₃(x). Then

\alpha _{{22}}\circ \beta _{{12}}(x_{{12}})=\beta _{{13}}\circ \alpha _{{21}}(x_{{12}})=\beta _{{13}}\circ \beta _{{03}}(x)=0,

i.e., β₁₂(x
₁₂) ∈ ker(α₂₂). By exactness of the second row, this means that β₁₂(x
₁₂) = α₂₁(x
₂₁) for some unique x
₂₁ ∈ K
₁ ⊗ M
₂. Then

\alpha _{{31}}\circ \beta _{{21}}(x_{{21}})=\beta _{{22}}\circ \alpha _{{21}}(x_{{21}})=\beta _{{22}}\circ \beta _{{12}}(x_{{12}})=0,

i.e., β₂₁(x
₂₁) ∈ ker(α₃₁). By exactness of the bottom row, this means that β₂₁(x
₂₁) = α₃₀(y) for some unique y ∈ Tor^Z
₁(L
₂, L
₁).

Upon checking that y is uniquely determined by x (not dependent on the choice of x
₁₂), this defines a function Tor^Z
_n(L
₁, L
₂) → Tor^Z
_n(L
₂, L
₁), taking x to y, which is a group homomorphism. One may check that this map has an inverse, namely the function Tor^Z
_n(L
₂, L
₁) → Tor^Z
_n(L
₁, L
₂) defined in a similarly manner. One can also check that the function does not depend on the choice of free resolutions.

References

Weibel, Charles A. (1994). An introduction to homological algebra. Cambridge Studies in Advanced Mathematics. 38. Cambridge University Press. ISBN 978-0-521-55987-4. MR 1269324. OCLC 36131259.

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

Tor functor

Definition

Properties

See also

References