Osculating curve

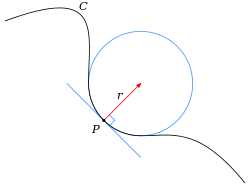
A curve C containing a point P where the radius of curvature equals r, together with the tangent line and the osculating circle touching C at P

In differential geometry, an osculating curve is a plane curve from a given family that has the highest possible order of contact with another curve. That is, if F is a family of smooth curves, C is a smooth curve (not in general belonging to F), and p is a point on C, then an osculating curve from F at p is a curve from F that passes through p and has as many of its derivatives at p equal to the derivatives of C as possible.[1][2]

The term derives from the Latinate root "osculate", to kiss, because the two curves contact one another in a more intimate way than simple tangency.[3]

Examples

Examples of osculating curves of different orders include:

  • The tangent line to a curve C at a point p, the osculating curve from the family of straight lines. The tangent line shares its first derivative (slope) with C and therefore has first-order contact with C.[1][2][4]
  • The osculating circle to C at p, the osculating curve from the family of circles. The osculating circle shares both its first and second derivatives (equivalently, its slope and curvature) with C.[1][2][4]
  • The osculating parabola to C at p, the osculating curve from the family of parabolas, has third order contact with C.[2][4]
  • The osculating conic to C at p, the osculating curve from the family of conic sections, has fourth order contact with C.[2][4]

Generalizations

The concept of osculation can be generalized to higher-dimensional spaces, and to objects that are not curves within those spaces. For instance an osculating plane to a space curve is a plane that has second-order contact with the curve. This is as high an order as is possible in the general case.[5]

See also

References

  1. 1 2 3 Rutter, J. W. (2000), Geometry of Curves, CRC Press, pp. 174–175, ISBN 9781584881667 .
  2. 1 2 3 4 5 Williamson, Benjamin (1912), An elementary treatise on the differential calculus: containing the theory of plane curves, with numerous examples, Longmans, Green, p. 309 .
  3. ↑ Max, Black (1954–1955), "Metaphor", Proceedings of the Aristotelian Society, N.S., 55: 273–294 . Reprinted in Johnson, Mark, ed. (1981), Philosophical Perspectives on Metaphor, University of Minnesota Press, pp. 63–82, ISBN 9780816657971 . P. 69: "Osculating curves don't kiss for long, and quickly revert to a more prosaic mathematical contact."
  4. 1 2 3 4 Taylor, James Morford (1898), Elements of the Differential and Integral Calculus: With Examples and Applications, Ginn & Company, pp. 109–110 .
  5. ↑ Kreyszig, Erwin (1991), Differential Geometry, Toronto University Mathematical Expositions, 11, Courier Dover Publications, pp. 32–33, ISBN 9780486667218 .
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.