Upper set

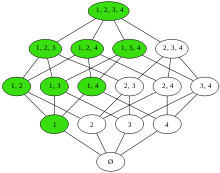
The powerset algebra of the set {1,2,3,4} with the upset ↑{1} colored green. The white sets form the downset ↓{2,3,4}.

In mathematics, an upper set (also called an upward closed set or just an upset) of a partially ordered set (X,≤) is a subset U with the property that, if x is in U and x≤y, then y is in U.

The dual notion is lower set (alternatively, down set, decreasing set, initial segment, semi-ideal; the set is downward closed), which is a subset L with the property that, if x is in L and y≤x, then y is in L.

The terms order ideal or ideal are sometimes used as synonyms for lower set.[1][2][3] This choice of terminology fails to reflect the notion of an ideal of a lattice because a lower set of a lattice is not necessarily a sublattice.[1]

Properties

  • Every partially ordered set is an upper set of itself.
  • The intersection and the union of upper sets is again an upper set.
  • The complement of any upper set is a lower set, and vice versa.
  • Given a partially ordered set (X,≤), the family of lower sets of X ordered with the inclusion relation is a complete lattice, the down-set lattice O(X).
  • Given an arbitrary subset Y of an ordered set X, the smallest upper set containing Y is denoted using an up arrow as ↑Y.
    • Dually, the smallest lower set containing Y is denoted using a down arrow as ↓Y.
  • A lower set is called principal if it is of the form ↓{x} where x is an element of X.
  • Every lower set Y of a finite ordered set X is equal to the smallest lower set containing all maximal elements of Y: Y = ↓Max(Y) where Max(Y) denotes the set containing the maximal elements of Y.
  • A directed lower set is called an order ideal.
  • The minimal elements of any upper set form an antichain.
    • Conversely any antichain A determines an upper set {x: for some y in A, x ≥ y}. For partial orders satisfying the descending chain condition this correspondence between antichains and upper sets is 1-1, but for more general partial orders this is not true.

Ordinal numbers

An ordinal number is usually identified with the set of all smaller ordinal numbers. Thus each ordinal number forms a lower set in the class of all ordinal numbers, which are totally ordered by set inclusion.

See also

  • Cofinal set – a subset U of a partially ordered set (P,≤) that contains for every element x of P an element y such that x ≤ y

References

  1. 1 2 Davey & Priestley, Introduction to Lattices and Order (Second Edition), 2002, p. 20 and 44
  2. ↑ Stanley, R.P. (2002). Enumerative combinatorics. Cambridge studies in advanced mathematics. 1. Cambridge University Press. p. 100. ISBN 978-0-521-66351-9.
  3. ↑ Lawson, M.V. (1998). Inverse semigroups: the theory of partial symmetries. World Scientific. p. 22. ISBN 978-981-02-3316-7.
  • Blanck, J. (2000). "Domain representations of topological spaces" (PDF). Theoretical Computer Science. 247: 229–255. doi:10.1016/s0304-3975(99)00045-6.
  • Hoffman, K. H. (2001), The low separation axioms (T0) and (T1)
  • Davey, B.A. & Priestley, H. A. (2002). Introduction to Lattices and Order (2nd ed.). Cambridge University Press. ISBN 0-521-78451-4.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.